Multiple Chromosomal Rearrangements Structured the Ancestral Vertebrate -Bearing Protochromosomes

Summary

While the proposal that large-scale genome expansions occurred early in vertebrate evolution is widely accepted, the exact mechanisms of the expansion—such as a single or multiple rounds of whole genome duplication, bloc chromosome duplications, large-scale individual gene duplications, or some combination of these—is unclear. Gene families with a single invertebrate member but four vertebrate members, such as the Hox clusters, provided early support for Ohno's hypothesis that two rounds of genome duplication (the 2R-model) occurred in the stem lineage of extant vertebrates. However, despite extensive study, the duplication history of the Hox clusters has remained unclear, calling into question its usefulness in resolving the role of large-scale gene or genome duplications in early vertebrates. Here, we present a phylogenetic analysis of the vertebrate Hox clusters and several linked genes (the Hox “paralogon”) and show that different phylogenies are obtained for Dlx and Col genes than for Hox and ErbB genes. We show that these results are robust to errors in phylogenetic inference and suggest that these competing phylogenies can be resolved if two chromosomal crossover events occurred in the ancestral vertebrate. These results resolve conflicting data on the order of Hox gene duplications and the role of genome duplication in vertebrate evolution and suggest that a period of genome reorganization occurred after genome duplications in early vertebrates.

Introduction

Ohno's hypothesis [1] that two-rounds of whole genome duplication (the 2R-model) occurred in the ancestor of extant vertebrates, over 450 million years ago (Figure 1A), has generally gained wide acceptance. Recently, however, the mechanisms of that genome expansion have been debated, with some studies finding strong support for two-rounds of whole genome duplication [2]–[7] while others have found support for a single round but not two rounds of whole genome duplication [8]–[10]; some authors have even questioned whether there is any support for whole genome duplications in the evolution of vertebrates [11]–[16]. Thus, even though there is wide support for 2R-model, the evidence for it is still conflicting. Central to this debate has been the duplication history of Hox clusters and associated linked genes (the “Hox paralogon”, Figure 1A/B). Although the chromosomal order of genes within the Hox paralogon vary within living gnathostomes [17]–[19], ancestrally the four vertebrate Hox clusters (HoxA-D) were closely linked to at least three other gene families such as Dlx, Col and ErbB. However, there is only a single cluster with associated linked genes in invertebrates [20]. This 1∶4 ratio has been used to support the most widely held version of the 2R-model in which two rounds of whole genome duplications were followed by extensive gene loss.

Hughes [14] has argued that gene families can be used to support two-rounds of whole genome duplication only if: 1) the “extra” vertebrate genes duplicated on the vertebrate stem-lineage and thus are vertebrate-specific paralogs, and 2) gene phylogenies show a symmetrical ((A,B)(C,D)) topology indicating two duplication events. Using these criteria, Hughes [14], Friedman and Hughes [11],[12] and Hughes, da Silva and Friedman [16] surveyed the duplication history of developmentally important genes, the Hox clusters and genes within the Hox paralogon (among others) and found that the majority of duplication orders were inconsistent with the ((A,B)(C,D)) topology, including the Hox clusters themselves, violating assumption 2. These authors concluded that there was little support for the 2R hypothesis and that genome duplications did not structure the Hox-bearing chromosomes, respectively (although see [21]).

The phylogenetic analyses of Hughes et al. [16] have been criticized on several grounds, particularly incomplete taxon and gene sampling [2]. In a detailed reanalysis of Hughes et al's data, Larhammar et al. [2] found that the majority of genes in the analysis were either recent tandem duplications or had multiple paralogs with complex translocation histories that made them unsuitable for inferring support of the 2R hypothesis. Of the remaining 20 families, the duplication history of 14 were consistent with 2R (for example ITGB, NHR and ACCN), while only 6 (AQP, ErbB, GLI, GNB, ITGA, NOS and SCN) had phylogenies that differed from the Hox clusters and contradict the 2R model. Larhammar et al. [2] concluded that available data were consistent with block/chromosome duplications and that the duplications likely occurred so rapidly that phylogenetic signal did not have time to accumulate, leading to the conflicting and poorly resolved gene phylogenies often cited as evidence against 2R.

Seriously confusing the debate is the duplication history of the Hox clusters themselves and the gene families most closely linked to the clusters (the “core” Hox paralogon), which have duplication histories that are apparently different from each other and the Hox clusters. For example, based on minimizing the cost of gene losses after cluster duplications, Kappen and Ruddle [22] found a single best tree with the order ((A,B)(C,D)), however, the next best tree with the order (B,(A,(C,D))) was only a single step away. The (B,(A,(C,D))) topology was also found by Zhang and Nei [23] using distance methods, but these authors could not reject an ((A,B)(C,D)) topology because internal branch support was low. The most detailed study of Hox cluster duplications and the duplication of the Hox-linked collagen (Col) genes found that likelihood, parsimony and distance phylogenetic inference methods and minimum-evolution branch length tests converged on the ((A,B)(C,D)) topology, but favored placing the root at the HoxD cluster leading to the duplication order (D(A(B,C))) [24].

Although there are only three paralog groups of the Dlx genes in most vertebrates, invertebrates have only a single group so the duplication history of Dlx genes can still contribute to the debate on which two clusters are most closely related. A detailed analysis of Dlx genes from multiple vertebrates, including shark, lamprey and invertebrates was consistent with a (D(B,A)) duplication order [25],[26]. While this topology cannot address the relationship of these genes with respect to the HoxC cluster, it suggests that clusters B and A are more closely related to each other than HoxD and a (D,C,(B,A)) topology.

At the other end of the paralogon (Figure 1), Hughes et al. [16] found that the duplication order of the ErbB genes strongly supported the (D(C(B,A))) topology, a duplication order not previously found in any previous analysis. Close examination of this data, however, indicates that the chromosomal location of HoxC/ErbB4 and HoxD/ErbB3 clusters was mislabeled (see Hughes et al. [16] Figure 4) and therefore the phylogeny of ErbB genes with respect to the Hox clusters was incorrect. The corrected topology of ErbB with respect to the Hox clusters is (C(D(B,A)). Again, a topology not previously supported.

Given these multiple conflicting topologies, it is clear that inferring the duplication history of Hox clusters and closely linked genes is extremely complicated. To clarify potential reasons for this uncertainty and resolve the duplication history of this region, we conducted a phylogenetic analysis of a core set of genes in the Hox paralogon (Dlx, Col, Hox and ErbB) that are closely linked and have no evidence of translocation to other chromosomes since the diversification of extant vertebrates. Our analysis indicates that there are two competing phylogenies that divide the members of the core Hox paralogon; Dlx and Col share a (D(C(A,B))) topology while Hox and ErbB share a (B(A(C,D))) topology. We suggest that these competing phylogenies can be resolved if two chromosomal rearrangements occurred after the clusters duplicated but before the diversification of extant vertebrates. Indeed, this scenario has been suggested to resolve incongruent branch orders of linked genes and supports the hypothesis that the ancestral vertebrate may have been pseudo-octoploid [18].

Results

We used several methods of phylogenetic inference because there are strengths and limitations to each method [27]. For example, neighbor-joining (NJ) and minimum evolution (ME) are widely-used, fast and perform well when divergence between sequences is low (like all methods), but a potentially serious limitation for these distance methods is that the inferred distances between genes may not accurately reflect the actual evolutionary distances between them. While compensating for variation in divergence rates can correct the inferred distances, as the degree of variation and divergence increase the effectiveness of corrections decrease [27]. Thus, when trying to infer older relationships, distance methods can fail or lead to strongly supported but incorrect results. Bayesian inference (BI) and maximum likelihood (ML) methods overcome these limitations by being based on an explicit model of nucleotide substitution that accounts for variation in evolutionary rates between nucleotide sites, but differ in how branch supports are assessed [27]. Like most other phylogenetic methods, ML use nonparametric bootstrapping to generate a confidence limit on branch supports. While widely used, bootstrap support values are generally conservative and underestimate true support when the signal-to-noise ratios are low [27]. On the other hand, BI uses the posterior distribution of trees sampled during the tree search to indicate branch support and reflect the probability the branch is correctly inferred given the data and the model; posterior probabilities generated from BI more accurately reflect branch support, but can be prone to over estimate confidence in clade support [27]. By utilizing multiple phylogenetic methods we can assess the impact of each methods assumptions on the resulting phylogeny, in addition congruence in the inferred topology between multiple methods can itself be taken as support for the topology [27].

Our phylogenetic analysis included: (1) HoxA-D clusters from human (Homo sapiens), chicken (Gallus gallus), frog (Xenopus tropicalis), coelacanth (Latimeria chalumnae), shark (Heterodontus francisci) HoxA and HoxD clusters and the single Amphioxus Hox cluster; (2) Col1A2, Col2A1, Col1A1 and Col3A1 genes from human (Homo sapiens), mouse (Mus musculus), dog (Canis familiaris), cow (Bos taurus) and chicken (Gallus gallus), Col2A1 and Col3A1 from frog (Xenopus tropicalis), and the single Collagen gene from Amphioxus; (3) Dlx1/2, Dlx6/5 and Dlx4/3 genes from human (Homo sapiens), mouse (Mus musculus), shark (Heterodontus francisci) and the single Dlx gene pair from Amphioxus, Saccolossus and Ptychodera; and (4) EGFR, ErbB2, ErbB3, and ErbB4 genes from human (Homo sapiens), mouse (Mus musculus), rat (Rattus norvegicus), dog (Canis familiaris), opossum (Monodelphis domestica), frog (Xenopus leavis) and the single ErbB gene of Ciona intestinalis. Teleost (bony fish) genes were not included because of the additional genome duplication in the stem-lineage of euteleosts.

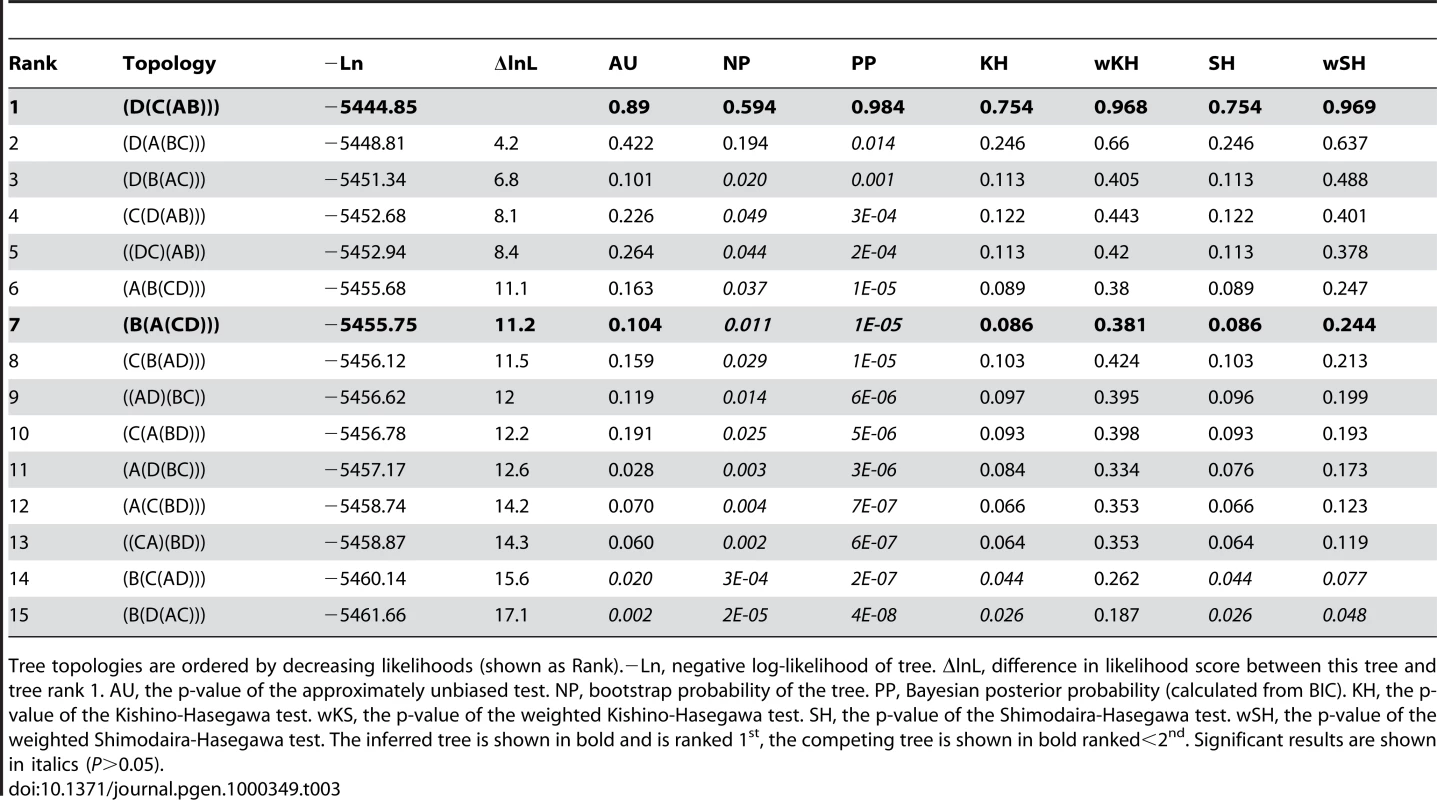
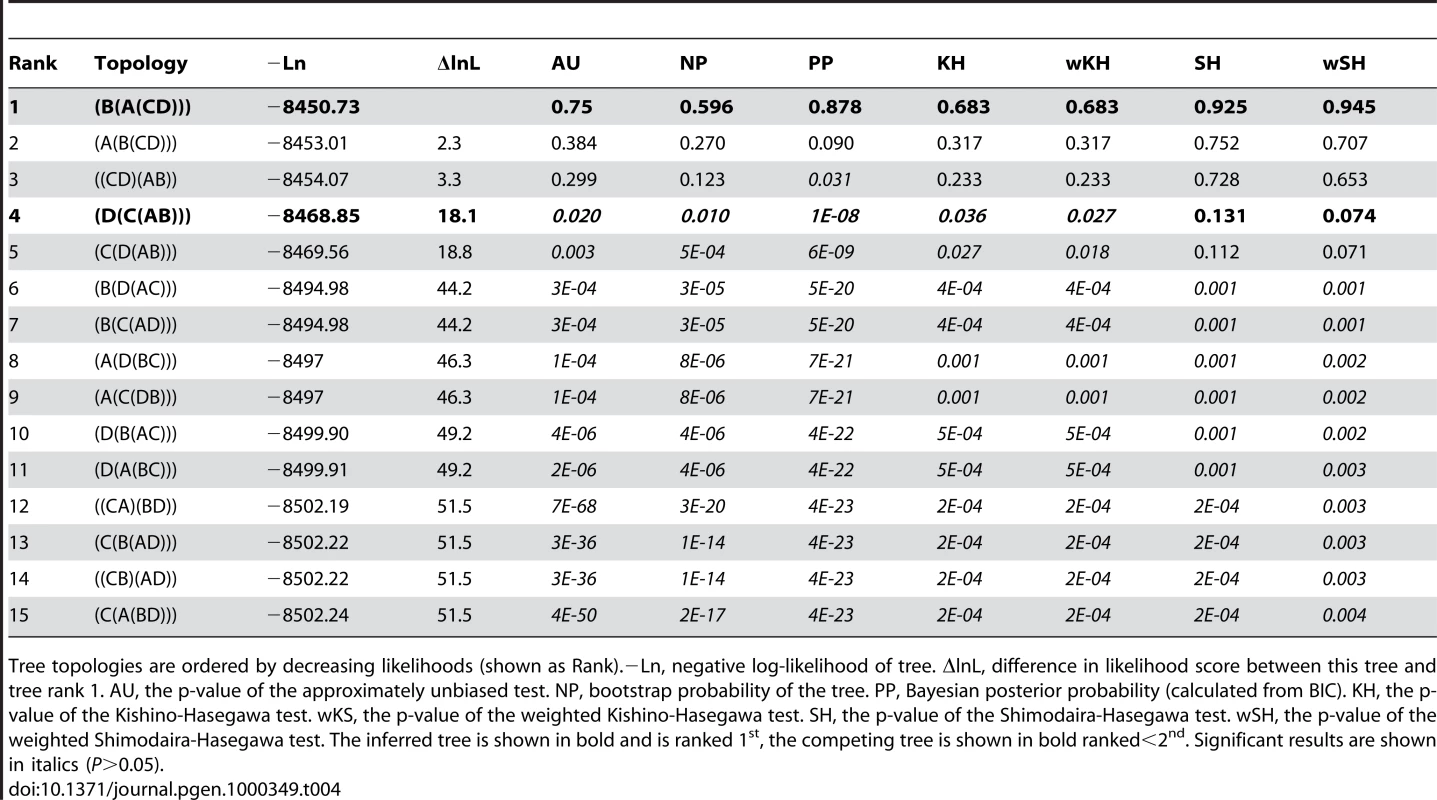
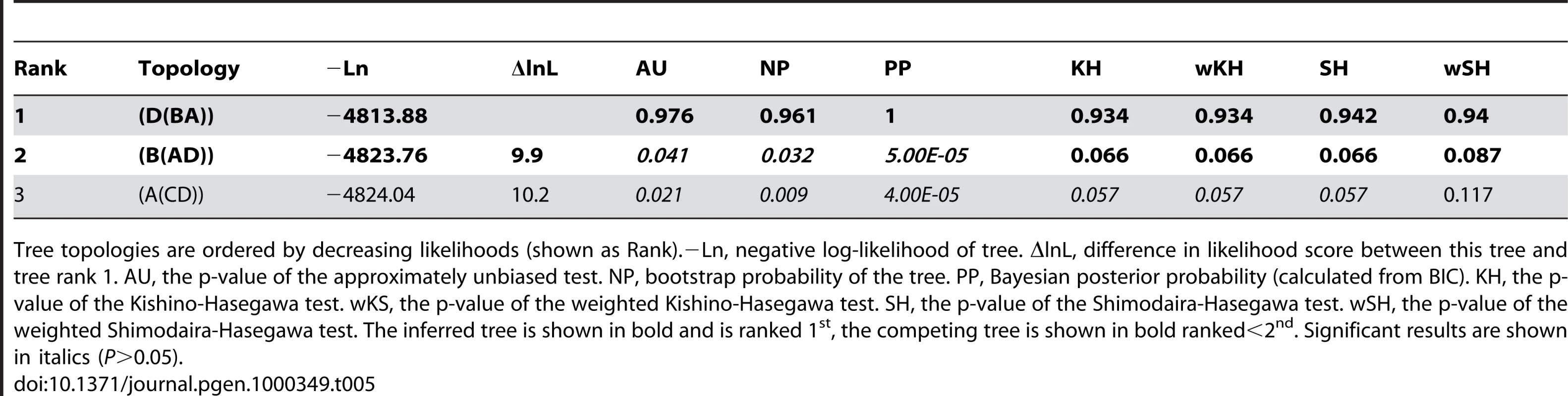
Phylogenetic analyses of the Hox, Col, Dlx and ErbB genes were performed using Bayesian inference (BI), maximum likelihood (ML), neighbor-joining (NJ) and minimum evolution (ME). Each method found the same topology for each gene with strong to moderate support (Figure 2). The most striking feature of the phylogenetic analyses was the split in inferred topologies between Dlx/Col and Hox/ErbB. Col genes support and Dlx genes are consistent with a (D(C(B,A))) duplication order while the Hox clusters and ErbB genes support a (B(A(C,D))) topology. Interestingly, the only difference between these two topologies is the location of the root; all genes converge on the unrooted topology (A,B(C,D)).

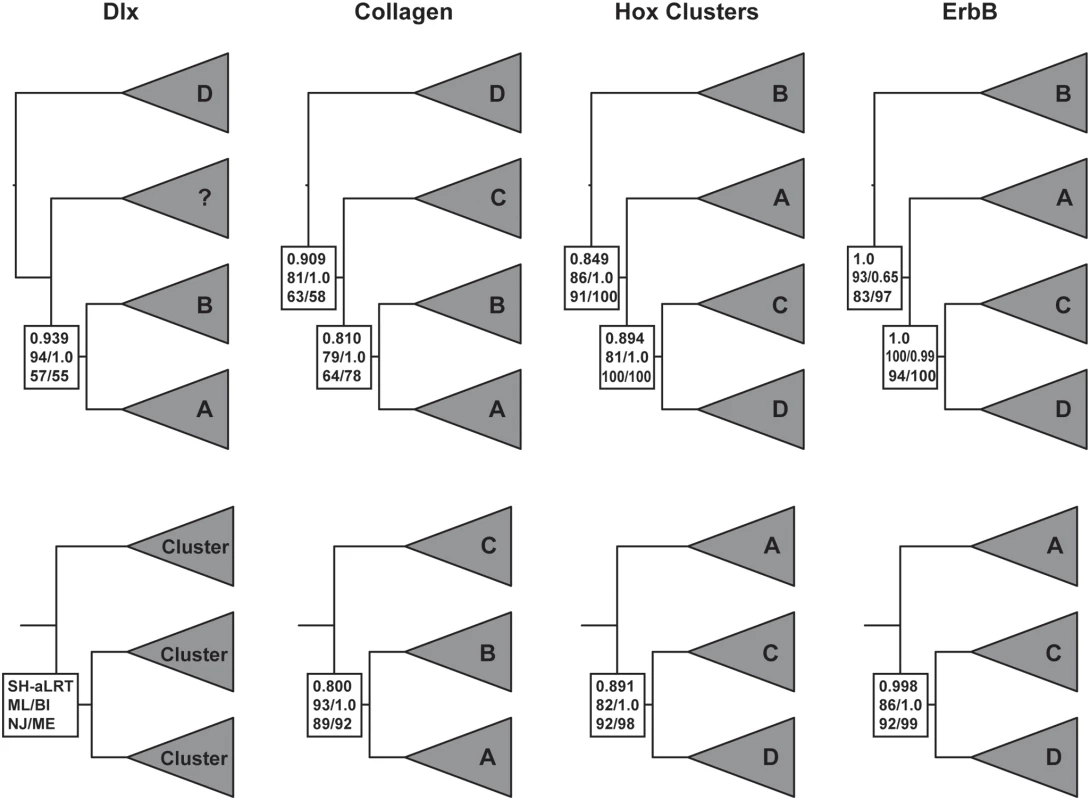
Several authors have noted that symmetrical ((A,B)(C,D)) topologies can be inferred as sequential (A(B(C,D))) because of long branch attraction, for example, if the out-group and one in-group clade evolve particularly fast [28]. This “pull of the past” artifact causes the most rapidly evolving in-group to cluster with the out-group solely because of homoplasy; when rooted by the out-group the resulting trees will not longer be symmetric [28]. Although problems of long-branch attraction (LBA) may be overrated [29] and do not appear to be effecting this data (see below), it is still a serious concern when genes that supposedly share a duplication history are inferred to have different phylogenies. One simple test is to remove the most basally placed in-group and re-infer the trees [29]. If the new in-group branching order is the same as the full dataset then long-branch attraction is unlikely to cause the sequential topology. Applying this test to the Col, Hox and ErbB data does not change the inferred branching order (Figure 2), thus long-branch attraction is unlikely to cause misplacement of the root. (Dlx was excluded from this analysis because there are only 3 vertebrate Dlx paralogs.)

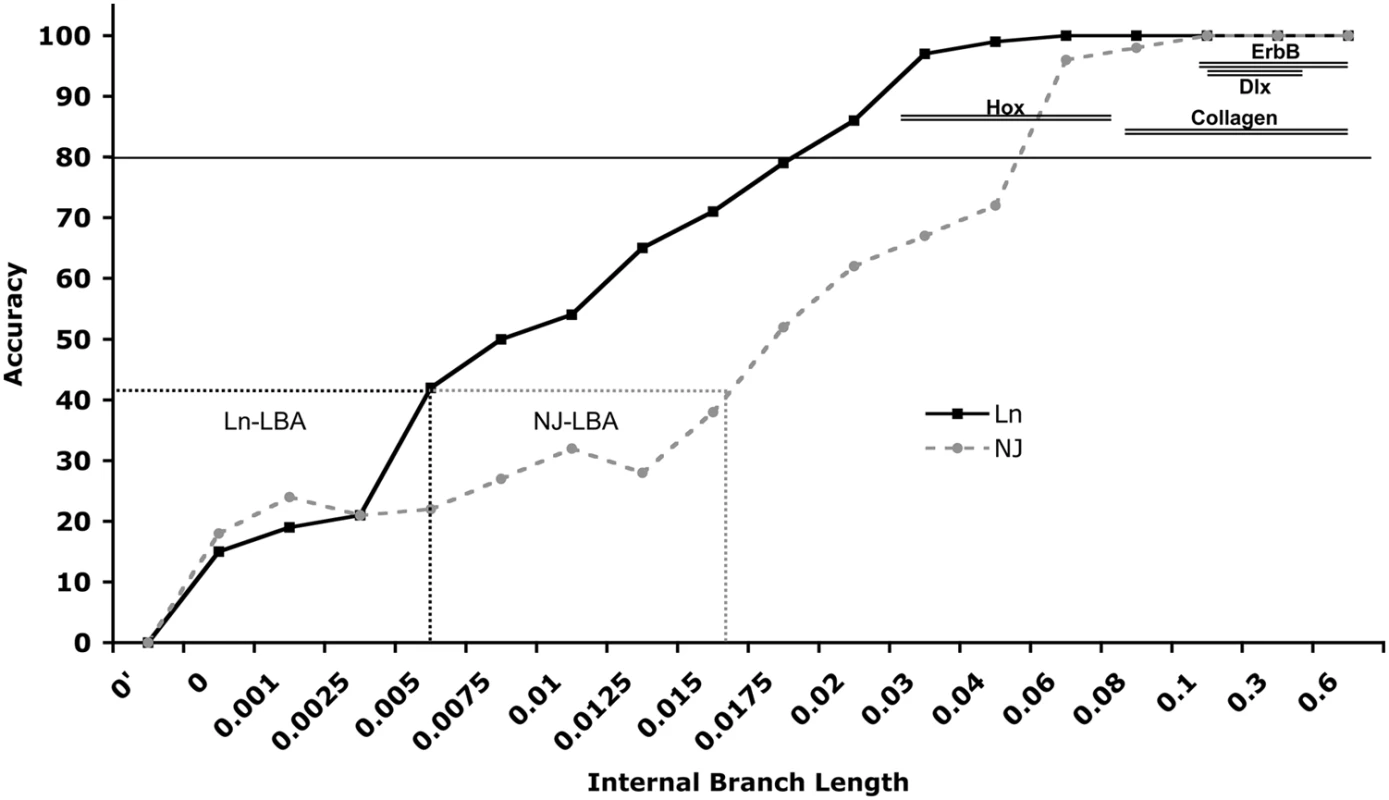
A potential problem with phylogenetic inference of lineages that split rapidly is that short branches can contain little phylogenetic signal and much noise [28]. This unfavorable signal-to-noise ratio can lead to erroneous tree inferences that are essentially rooted randomly but with strong support [28]. Similarly, rooting trees using an out-group has been shown to produce incorrect trees when the in-group internal branch lengths are short [28]. We examined the effect of branch lengths on tree topology by simulating datasets with a (B(A(C,D))) topology and increasing internal branch lengths. These simulated datasets were used for ML and NJ tree inference to find the internal branch-length at which tree support collapses or becomes misleading.

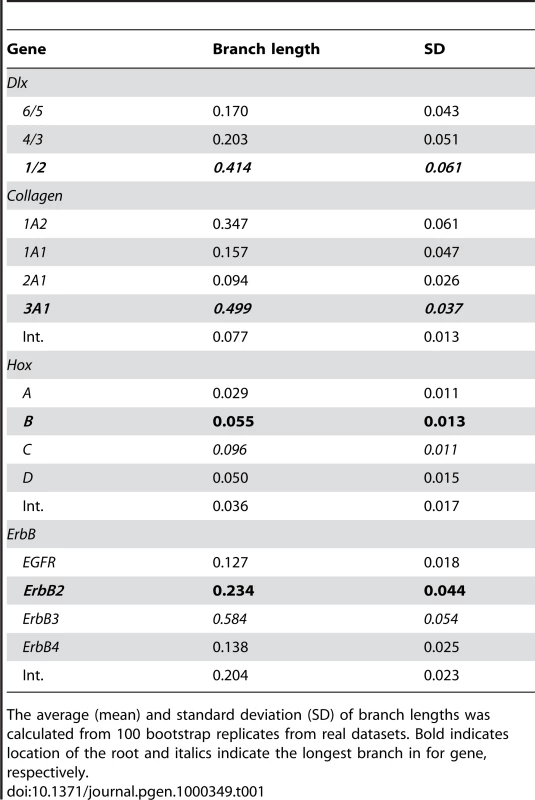
The results of the branch-length simulations (Figure 3) indicate that at extremely short internal branch lengths (0.001–0.0025), the root is consistently misplaced at the base of the C clade (∼43/100), which is the longest branch in both the real and simulated data (Table 1) along with the outgroup, indicating misplacement results from long branch attraction. At short internal branch lengths (0.005–0.015) the root is placed at C less often, but still at high frequency (∼28/100). At moderately short branch lengths (0.0175–0.02), however, there is a dramatic decrease in the frequency of trees rooted at C (∼5/100) indicating little detrimental effects from LBA. Above branch lengths of 0.025 no trees are misrooted at C and the majority are rooted correctly indicating no LBA artifacts. Thus, branches of length >0.0175 should be free, or nearly so, of long-branch attraction artifacts and other errors associated with random rooting at short internal branches. Indeed, the length of the internal branches (B(A,C,D) and (B,A(CD)) for Dlx, Col, Hox and ErbB genes are significantly greater than 0.0175 (Table 1), indicating the LBA and misrooting are unlikely causes of the divergent rootings.

A recently developed branch support measure, the approximate Likelihood Ratio Test (aLRT), can also be used to assess the support for branches [30]. This method compares the likelihood of a tree with the branch of interest collapsed to alternate model in which the branch has the length inferred from the data and tests whether there is sufficient data for the inferred branch to be “real”. Results from the Shimodaria-Hassegawa-like aLRT (a more conservative measure than the χ²-based aLRT) indicate there is strong support for each branch, particularly for post-duplication lineages (Figure 2). The more liberal χ²-based aLRT supported each branch with >0.95 values.

We also explicitly tested the location of the root for Dlx, Col, Hox and ErbB genes using parametric bootstrapping [31]. For each gene family we used the model of nucleotide substitution selected for the phylogenetic inference to simulate 100 replicate datasets on phylogenies with the alternate root, i.e. rooted at B for Dlx/Col and rooted at D for Hox/ErbB. These simulated datasets were then used to infer trees with ML, NJ and ME. If systematic biases in the data are responsible for the difference in rooting between Dlx/Col and Hox/ErbB then trees inferred from these simulated data should be incorrect (i.e. the root should be placed at some other internal branch). The correct tree, however, was inferred for all genes and methods in 94–98/100 replicate datasets, further indicating that these inference methods/data are robust to long-branch artifacts and systematic error/bias.

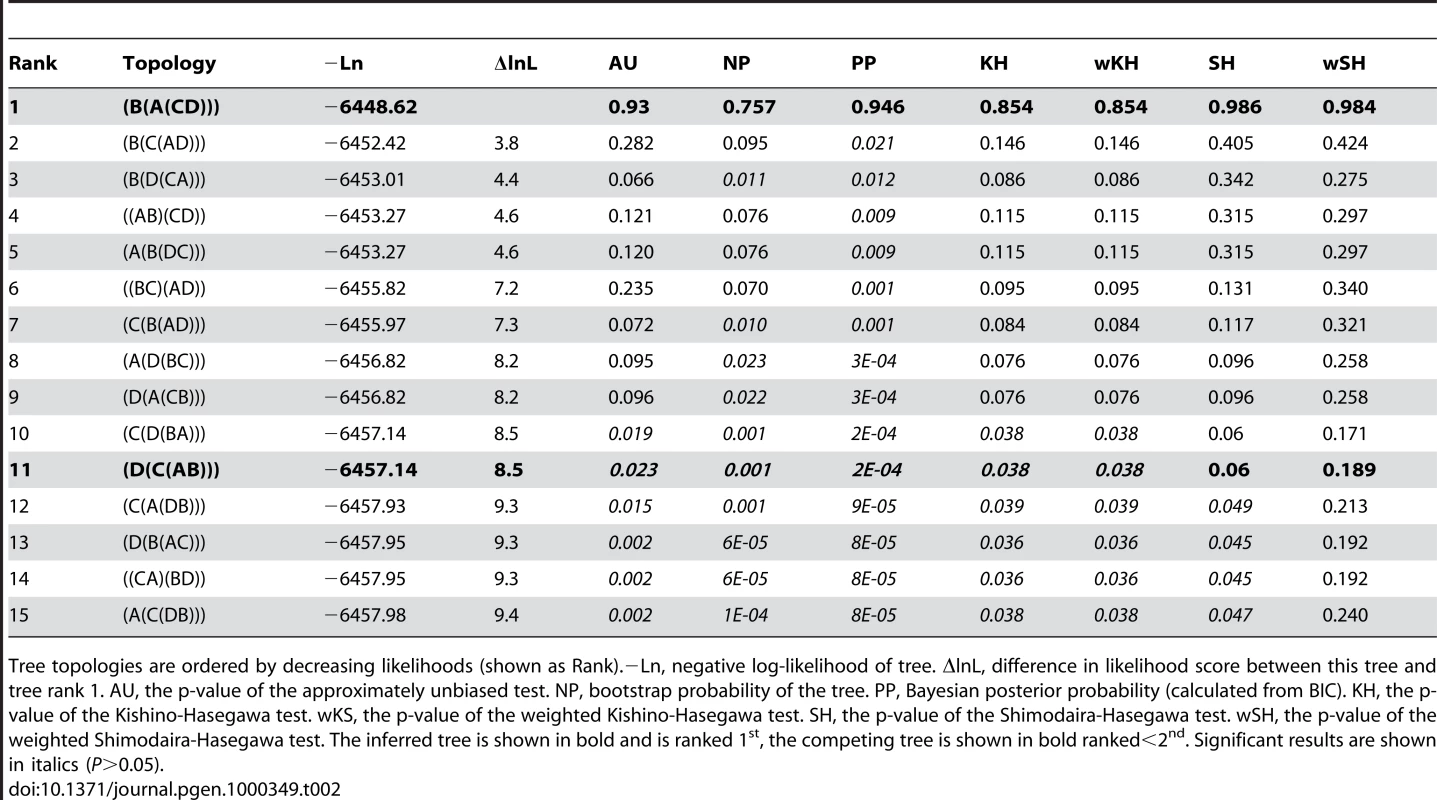
Finally, we tested alternate roots for Dlx, Col, Hox and ErbB genes using several methods implemented in the program CONSEL [32] that determine the confidence in the inferred tree by examining P-values for a set of alternate trees. The P-values of the Approximately Unbiased (AU) test [33], the Bootstrap Probability (NP) [32], the Bayesian Posterior Probability (PP) [32], the Kishino-Hasegawa (KH) test [34], the weighted Kishino-Hasegawa (wKH) test [32], the Shimodaira-Hasegawa (SH) test [35], and the Weighted Shimodaira-Hasegawa (wSH) test [32] were inferred for each possible rooting and in-group topology (15 alternate trees for Col, Hox, and ErbB; 3 alternate for Dlx). Like phylogenetic methods, these methods of selecting between competing phylogenetic tress each have strengths and weaknesses. For example, the AU and SH tests account for biases in selecting competing trees that are overlooked in the bootstrap probability and KH tests [33]. These corrections can lead to a more robustly supported tree, but can also make them too conservative [33]. By comparing the “best” tree scored by several methods (given as P-values), the effect of each methods assumptions on selecting the “best” tree can be ascertained. For each gene family we studied, the inferred root was significantly better than alternate rootings by most, if not all, of the selection methods indicating that method assumptions had little effect on picking the best tree (Tables 2–5).

Discussion

Nearly 40 years after Ohno first proposed that the vertebrate genome evolved through two successive rounds of whole genome duplication [1], the role of large-scale gene, chromosome and/or whole genome duplications in vertebrate genome evolution remains controversial. While the exact mechanisms of genome expansion are debated, there is now little doubt that expansion occurred. Analysis of human paralogs, for example, indicates that both large- and small-scale duplications played an important role in vertebrate genome evolution, with many of the duplications occurring in large blocks (en bloc) of chromosomes or chromosome segments [2],[8]. These duplication events occurred in at least three waves, the largest of which occurred in the early stages of vertebrate evolution coincident with expectations of the 2R model [8].

The Hox clusters have played a central role in the genome duplication story, largely because they conform to the 1∶4 expectation of the 2R hypothesis and are tightly linked to each other and several non-Hox genes. However, numerous studies of the duplications of the Hox clusters and linked genes have failed to reach a consensus on the mechanisms, number and order of duplications [2]–[16], [21]–[26],[36]. Many of these studies were hampered by limited sequence data and poor taxon sampling, lack of appropriate out-group data or computational limitations that prevented the use of computationally intensive methods of phylogenetic inference (such as Bayesian inference and maximum likelihood). Given these difficulties it is not surprising that nearly every study found support for a different duplication order.

Our analyses of the Dlx, Col, Hox cluster, and ErbB gene duplication histories identified an unexpected pattern that divides the core paralogon into two clear topological regions: the Dlx/Col region supporting a (D(C(B,A))) branching order while the Hox/ErbB region supports an alternate rooting of (B(A(C,D))). The topology of each region is moderately- to highly-supported by nonparametric bootstrap support values from multiple methods of phylogenetic inference (ML, NJ, ME) and highly supported by Bayesian posterior probabilities; this congruence of topologies among methods can itself be taken as a strong indicator of tree accuracy [37].

Trees that differ only in the placement of the root are generally thought to arise because of out-group misplacement either from LBA artifacts, other kinds of systemic bias or short internal branch lengths [28],[29]. Our numerous tests, however, indicate that root misplacement is not likely to be the cause of the two different topologies found for genes in the Hox paralogon. Indeed, these topologies appear to be particularly robust to the kind of systemic error and bias that would cause out-group misplacement. Thus, we conclude that the split in duplication history is likely to be real and results from two chromosomal rearrangements that occurred between the Col genes and Hox clusters after the duplication events but before the radiation of extant vertebrates.

Interestingly, our finding of structural changes in the Hox paralogon bearing chromosomes following the ancestral vertebrate genome duplication and recombination breakpoints between human Hox paralogon members (see below) may shed light on previous findings of differential molecular evolution in anterior (3′) and posterior (5′) Hox genes [38],[39]. Several studies have shown that the rate of molecular evolution is not uniformly distributed across the genome, with genes evolving faster near genomic regions with high recombination rates than genes near regions with low recombination rates [40],[41]. The findings that posterior Hox genes evolve faster than anterior and middle Hox genes within gnathostomes (termed Laxitas terminalis) [38], between phyla and subphyla (termed Posterior Flexibility) [39] and after genome duplications [42] may reflect this general trend.

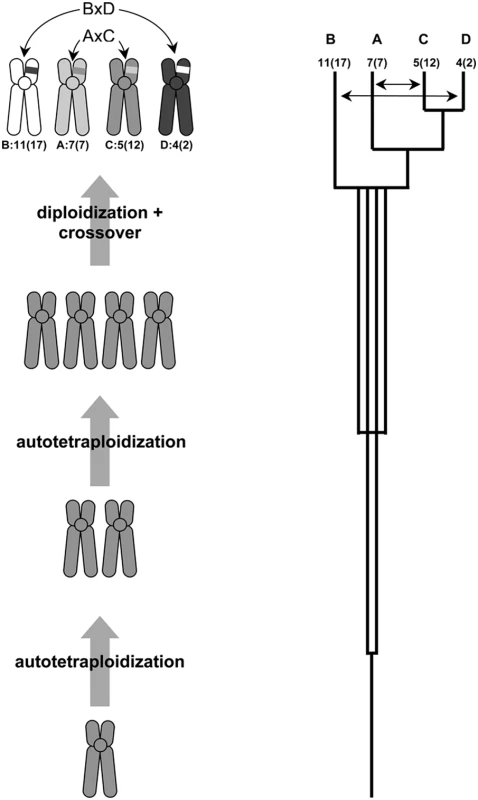
Furlong and Holland [18] have argued that asymmetrical trees and incongruent topologies between linked genes are not evidence against whole genome duplications as some have argued [11]–[16],[43], but are in fact a prediction of the 2R-model if both duplications occurred by rapid autotetraploidy. For example, if the diploidization after the first genome duplication (tetraploidization) was nearly complete by the time of the second duplication event, then gene trees would be sequential such as (A(B(CD))); during this pseudo-octaploid phase crossovers are likely to occur, because sequence divergence between homologous regions is still relatively low, resulting in gene trees that are incongruent between linked genes. However, given that sequence similarity is low enough to allow recombination, how is it possible to have relatively strong phylogenetic signal? The answer to this paradox likely lies in the evolution of Hox genes after duplications. For example, positive selection acting on Hox genes after the cluster duplications in teleost fish actually generated strong phylogenetic signal by rapidly fixing amino acid substitutions that preserved information on the duplication history [44]. Simiarly, positive selection acted on the Hox genes immediately after cluster duplications in vertebrates, rapidly fixing amino acid substitutions [42] and likely preserving a phylogenetic footprint of duplication order.

Our data suggest that at least two chromosomal crossover events occurred between the vertebrate protochromosomes bearing the core Hox paralogon genes, but are such chromosomal rearrangements likely? Several studies have shown that large- and small-scale chromosomal rearrangements are common after whole genome duplications [45]–[48], indicating that rearrangement of the vertebrate protochromosomes was extremely likely. For example, chromosomal rearrangements occurred within a few generations of hybridization in allotetraploid crosses of Arabidopsis suecica and Arabidopsis thaliana [47] and are common in autotetraploid Salmonid fish [49]. Inferring the pattern of chromosomal rearrangement after the vertebrate genome duplications may not be possible at a fine scale, but clues to the frequency of crossover events involving the core Hox paralogon genes can be found in a recent map of recombination rates in the human genome [50]. Remarkably, several windows of high recombination rate are found between genes in the paralogon (Figure 4). While these regions of high recombination rate indicate that crossovers occur between homologous chromosomes bearing the Hox paralogon in humans, they can only suggest that similar processes were at work after the whole genome duplications in the vertebrate ancestor.

Conclusions

The pattern of gene duplications for the core Hox paralogon genes is best explained by the proposal of Furlong and Holland [18] and provides a convincing case of a chromosomal crossover event between vertebrate protochromosomes 11 and 4, and 7 and 5 over 450 MYA (Figure 5)[51]. Most importantly, the identification of these chromosomal rearrangements in a highly conserved vertebrate syntenic block reconciles the conflicting interpretations of gene trees for this region and supports the hypothesis that large scale chromosomal or whole genome duplications contributed to vertebrate genome evolution. Further, these results support the proposal of Furlong and Holland [18] that the duplication events were the result of autotetraploidy, and that vertebrates are pseudo-octaploids.

Materials and Methods

Phylogenetic Analysis

Genes for Dlx, Collagen (Col), Hox homeodomains, and ErbB were downloaded from GenBank or identified from BLAST searches of nucleotide and amino acid databases; the alignments are available from VJL by request. The homeodomains of all paralog members for each Hox cluster were concatenated into a single alignment. Amino acid sequences for all genes were aligned with MUSCLE [52],[53] and adjusted by eye. Regions with large gaps, ambiguous alignment or repetitive sequences were removed from all genes.

Appropriate models of sequence evolution were estimated for each dataset with the program ModelGenerator [54] with the gamma rate parameter (approximated with 4 rate categories) and the proportion of invariable sites estimated from the data where appropriate. Phylogenetic trees were reconstructed using neighbor-joining (NJ), distance (minimum evolution), and maximum likelihood (ML) algorithms implemented in the PHYLIP v3.6 package of programs. ML trees were also generated using PhyML v2.4 [55]. (There were no significant differences between these implantations of ML and results from PhyML are reported.) Branch support was assessed with 1000 bootstrap resamplings for NJ, distance, and ML. The approximate likelihood ratio test implemented in PhyML was also used to infer branch support for ML trees. Bayesian trees were generated with Mr.Bayes v3.0 [56], running 2 sets of 4 chains for 10,000,000 generations sampling every 1000^th tree. Convergence of model parameters was assayed using Tracer (http://tree.bio.ed.ac.uk/software/tracer/) and ensuring the average standard deviation of split frequencies was less than 0.01.

Tests of Tree Topologies

The 15 alternate rootings of Hox, Collagen and ErbB genes, and the 3 alternate rootings of the Dlx genes were directly tested to determine if the roots inferred from phylogenetic analyses were significantly better than all alternate roots using the methods implemented in the program CONSEL [32]. Parametric bootstrap tests were also used to test for the effects of rooting of Collagen/Dlx at HoxB (these genes are inferred to be rooted at HoxD) and Hox/ErbB at HoxD (these genes are inferred to be rooted at HoxB). 100 replicate datasets were generated using Seq-Gen (http://tree.bio.ed.ac.uk/software/seqgen/) for each gene family using a model of evolution that matched the model inferred from the real dataset and a tree topology that changed the location of the root. Similarly, to test for the affect of branch lengths on the inferred tree topology, 100 replicate datasets were generated using Seq-Gen for each branch length set using a model of evolution (similar to that inferred for the Hox gene dataset). Internal branch lengths in the model tree (from which Seq-Gen generated simulated datasets) were incrementally increased from 0–2. Finally, the effect of root misplacement and branch-length on the accuracy of tree inferences was examined by inferring trees from each replicate dataset with ML and counting the frequency of that the true tree was inferred or plotting the branch length against the average internal branch support (shown in Figure 3).