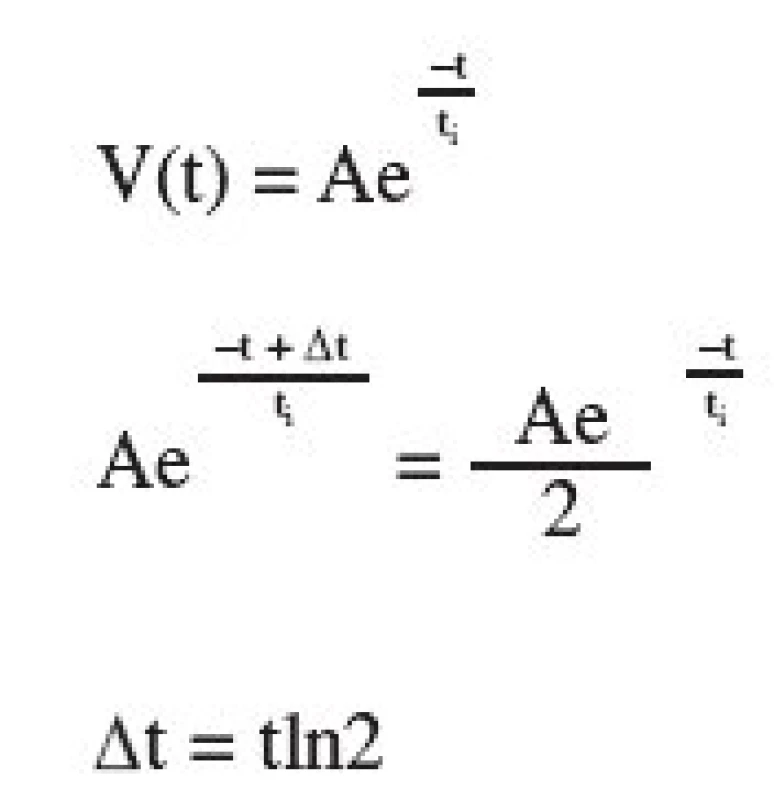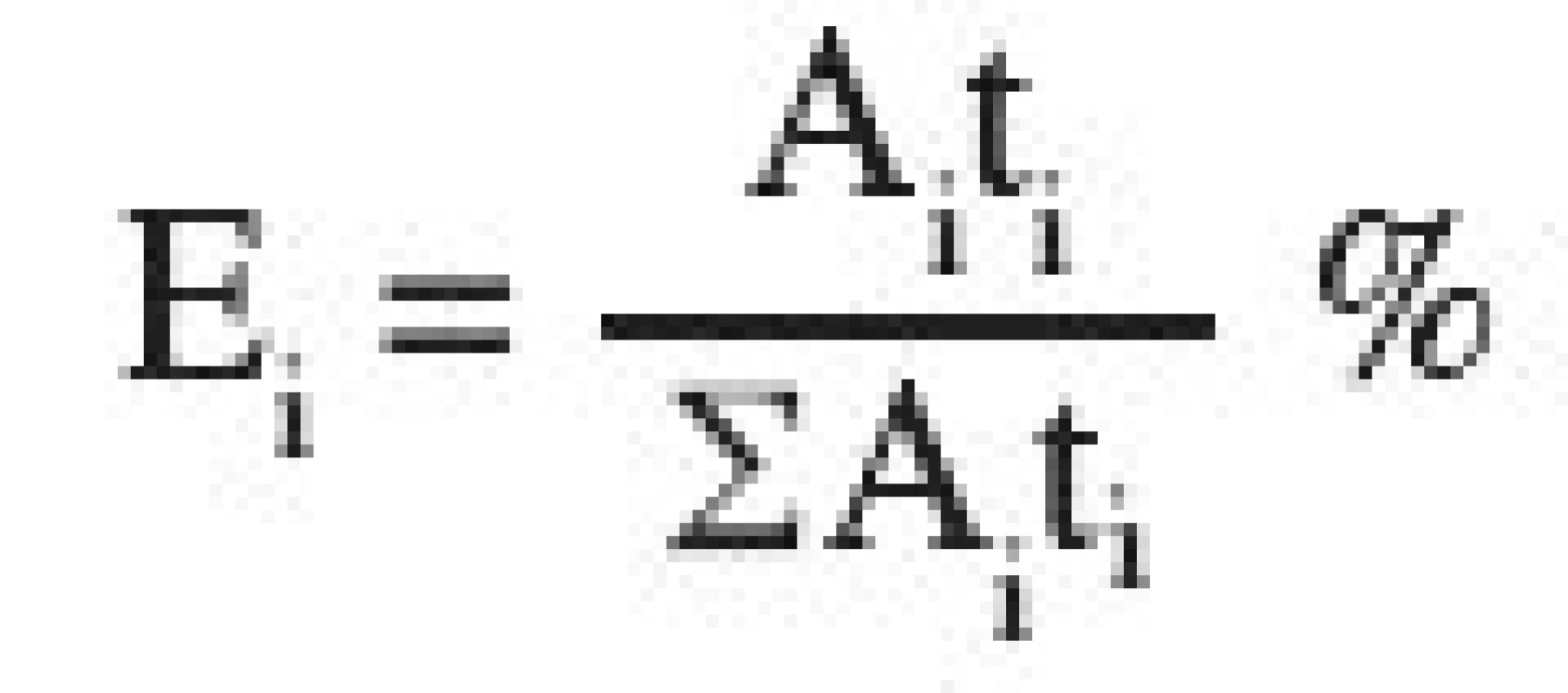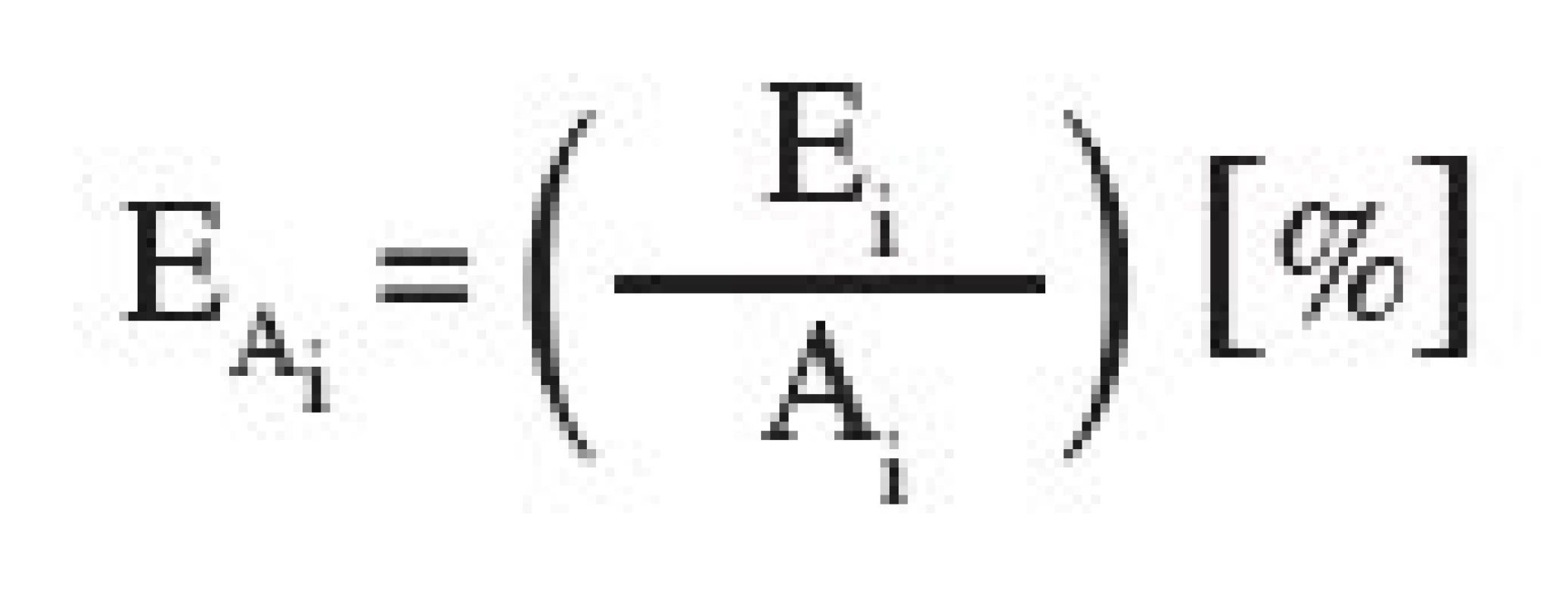Characterization of microcrystalline celluloses by means of the parameters of a three-exponential compression equation

Overview

The study compares microcrystalline celluloses by means of the parameters of the three-exponential equation V/V₀ = A₁*exp(-P/t₁) + A₂*exp(-P/t₂) + A₃*exp(-P/t₃) + y₀¹). The process of compression, according to this equation, takes place in three stages: in the stage of reduction of interparticular pores, reduction of intraparticular pores, and in the stage of reduction of the solid substance without pores. The classic theory of compression is based on the fact that the individual processes take place within the ranges of concrete compression pressures. According to the new equations, the processes under evaluation take place concurrently within the whole range of compression pressures employed. The paper studied four microcrystalline celluloses, Avicels PH 102, PH 103, PH 105, and PH 301. These excipients differ in sizes and shapes of particles, density, and content of humidity. The evaluations included the sizes of reductions A_R, sizes of energies E_A, and “half-pressures” P_H. The results of the study show that with diminishing particles of microcrystalline celluloses energies E_A are decreased and “half-pressures” are simultaneously increased. Best compressibility was found in Avicel PH 102 with the value of the “half-pressures” of 119.954 MPa and the worst in Avicel PH 301 with the value of the “half-pressures” of 151.449 MPa.

Key words:
three-exponential equations of compression – microcrystalline celluloses – theory of compression of tablets

Introduction

The compression process is characterized by the compression equation. It expresses the effect of compression pressure on the changes in the height, volume, or density of the tablet. At present the research of tablets uses three models of compression equations. The first and oldest model is the expression of the compression process by a straight-line equation. This model is represented by the equation according to Walker ²⁾ and Balshin ³⁾. The second model expresses the compression process by the hyperbolic equation, an example being the equation according to Kawakita and Lüdde ⁴⁾. The third model is the expression of the compression process by means of a two-exponential equation. This method was employed by Cooper an Eaton ⁵⁾. This equation was created for the compression of ceramic materials. It has been found that the above-mentioned equation describes the compression process of crystalline pharmaceutical excipients, but it is not suitable for polymeric excipients. The three-exponential equation is more suitable for these substances:

Vo is the initial volume of the tableting material which is being compressed, V, the volume of the tableting material at a given compression pressure. The parameter y represents the volume of the compact V_∞at the endless compression pressure. P are the compression pressures. Parameter A₁ represents the reduction of interparticular pores, parameter A₂ the reduction of intraparticular pores, and parameter A₃ the reduction of the solid substance without pores. Parameters 1/t_1–3 are the velocity constants of the appropriate reductions of volumes, out of which other parameters P_H1–3 are calculated. These parameters express the compression pressures, up to which one half of the reduction of interparticular pores, intraparticular pores, or the reduction of the solid substance without pores take place. The new parameters E_1–3 express the energy consumed to materialize the given reductions of the volume. The parameters E_A1–3 express the energy related to a unit of parameter A, i.e. a unit of the reduction of volumes in individual processes.

The study included the microcrystalline celluloses Avicel PH 102, PH 103, PH 105 a PH 301.

Experimental PART

Raw materials employed

The model dry binders for direct compression were the microcrystalline celluloses Avicel PH102, PH 103, PH 105, PH 301 manufactured by the firm FMC Corporation, Belgium. All materials were Ph. Eur. grade and used as purchased without preconditioning.

Preparation of tablets

Tablets of the diameter of 13 mm and weight of 500 mg were compressed in a compression preparation (Adamus HT, Machine Factory Group, Szczecin, Poland) in an apparatus for testing tensile and compression strengths T1-FRO 50 (Zwick GmbH, Ulm, Germany). Tablets were compressed using the following adjustment of the apparatus: distance of the punches 115 mm, standard strength 42000 N, unloading of the cycle 10 mm, velocity of the cycle 2 mm/s, pre-loading 2N, and pre-loading velocity 2 mm/s at the following compression forces: 50N, 100N, 150N, 200N, 250N, 300N, 350N, 400N, 450N, 500N, 750N, 1000N, 1500N, 2000N, 2500N, 3000N, 3500N, 4000N, 4500N, 5000N, 5500N, 6000N, 6500N, 7000N, 7500N, 8000N, 8500N, 9000N ,9500N, 10000N, 11000N, 12000N, 13000N, 14000N, 15000N, 16000N, 17000N, 18000N, 19000N, 20000N, 22000N, 24000N, 26000N, 28000N, 30000N, 32000N, 34000N, 36000N, 38000N, 40000N. In each of the compression forces 20 tablets were evaluated.

Evaluation of the parameter of the compression process

The following three-exponential equation of compression was employed to calculate the parameters of the compression process:

The parameters of the above-mentioned equations were calculated by means of the programme OriginPro 7.5 by the function ExpDec3. The sum of parameters A_1–3 and y represents the total volume of the compressed material at zero compression pressure. Parameter y represents the volume of the solid substance at endlessly large compression pressure. For a better comparison of the individual excipients, the sum of the reductions A₁, A₂ and A₃ was converted to the value of 1.0, thus obtaining parameters A_R1, A_R2 and A_R3. Volume changes in all tested substances thus ranged between 0 and 1. After this modification, the following equation was obtained:

Values t_1, t_2, t₃ represent changes in compression pressure which cause a change in the volume V/V to a certain value. These parameters were employed to calculate the “half-pressures” and the energy of compression.

Values t_1, t_2, t₃ served to calculate parameters P_H1, P_H2, P_H3. These new parameters, “half-pressures”, express the compression pressures at which reductions of interparticular pores, intraparticular pores, or reduction of a solid substance without pores to one half take place.

New parameters were calculated according to the following equations:

The following relations were employed to calculate the percentages of the energies of individual processes from parameters t_1, t_2, t₃:

dV = V’(P)dP

dE = P.V’(P)dP

This results in the conclusion that there is a dependence of the travel on force, but because the resultant energy is expressed in percents and calculated from relations (see the relation shown below), the percentual representation of energy for each process is identical also as far as the dependence of the ratio volume V/V on compression pressure P is concerned.

The calculation of the percentage of energy for each process uses the equation:

Energies E_{A 1–3} related to reductions of volumes were calculated according to the equation:

Results and discussion

Due to their good compressibility, microcrystalline celluloses are widely used excipients in the capacity of a filler for direct compression or a binder for most granulation ⁶⁾.

Cellulose fibres are composed of a large number of hollow microfibres. Two segments are recognized in these microfibres. There is a paracrystalline region, which is amorphous mass of cellulose chains, and a crystalline region, which is composed of solid bundles of cellulose chains in a solid linear arrangement. In their manufacture, as a result of acid hydrolysis, the amorphous region is partly removed to the detriment of the crystalline region ⁷⁾.

The binding properties are based on the hydrogen bridges between hydroxyl groups of the material. By means of compression pressure, the particles get into a very close contact and thanks to it these bonds develop very readily and on a large area. This is the reason for very good compression properties of microcrystalline cellulose and its good strength. Compression properties of microcrystalline cellulose are also influenced by humidity. The largest amount of water molecules is contained in the porous structure of microcrystalline cellulose and a certain share of moisture plays an important role in the formation of hydrogen bonds in these materials ⁸⁾.

Four microcrystalline celluloses were evaluated in the study. The obtained results are presented in Tables 1–3 and Figs. 1–5. The principal microcrystalline cellulose for the evaluation was Avicel PH 102. It possesses an average particle size of 100 μm, humidity 5%, and density D_MAX 4.756 g/cm³. This substance possesses the parameters A_R1 0.194, A_R2 0.444 and A_R3 0.361 and E_A1 0.032%, E_A2 0.209%, E_A3 2.492% and the “half-pressures” P_H1 1.539 MPa, P_H2 10.063 MPa and P_H3 119.954 MPa.

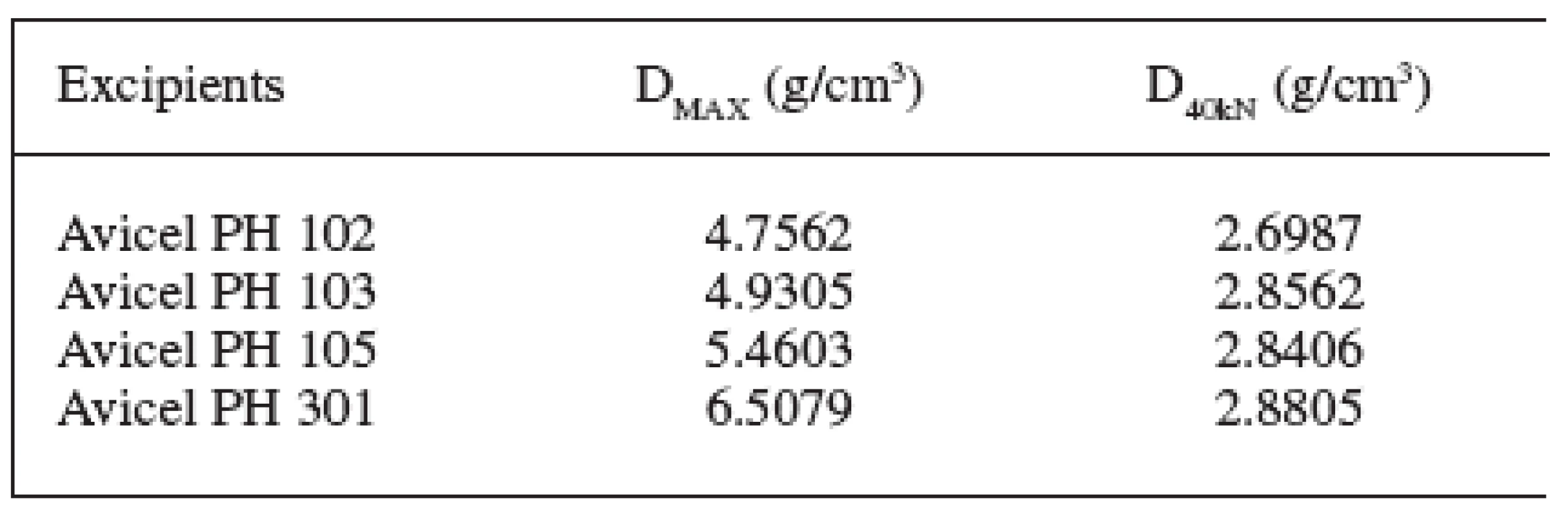
Avicels PH 103 and PH 105 in comparison with Avicel PH 102 possess an approximately identical density D_MAX, but they differ in the average particle size. Avicel PH 103 has the size of 50 μm and Avicel PH 105 20 μm. Reductions of interparticular pores between three Avicels under comparison are approximately identical. However, the evaluation of other two parameters is different. With increasing particle size the reduction of intraparticular particles is increased and at the same time the reduction of the solid substance without pores is decreased. The parameters of reductions of volumes are related directly to particle size and thus the pertinent relations after poring of the excipient into the matrix. With decreasing particle size, energies E_A are decreased in all three stages of the compression process. It seems that the bonds between surface chains of cellulose consume less energy than those between the chains within the particles. The final criterion of evaluation is “half-pressures”. In the stage of reduction of interparticular pores, “half-pressures” in all three microcrystalline celluloses are approximately identical in the range from 1.199 to 1.538 MPa. But in two subsequent stages the “half-pressures” are increased with the decreasing particle size, in the stage of reduction of intraparticular pores in the range from 10.063 to 12.509 MPa and in the stage of reduction of the solid substance without pores in the range from 119.954 to 137.437 MPa. It means that with the diminishment of the particles of microcrystalline celluloses energies E_A are decreased with a concurrent increase in the “half-pressure” values.

Another microcrystalline cellulose under evaluation is Avicel PH 301. It differs from Avicel PH 102 in a substantially higher density D_MAX at the level of 6.507 g/cm³. Its particle size is 50 μm and humidity content, 5%. In contrast to Avicel PH 102, it has lower values of reductions of both interparticular and intraparticular pores, which may be caused by its particle size. On the other hand, the reduction of the solid substance without pores is higher, caused probably also by a higher density. Energies E_A in Avicel PH 301, in contrast to Avicel PH 102, are in all three stages of compression process lower and “half-pressures” higher.

An important criterion of evaluation is the values of “half-pressures”. Lower values in the stage of reduction of the solid substance without pores mean better compressibility. From the given aspect, the best compressibility is found in Avicel PH 102 with the “half-pressure” value of 119.954 MPa and the worst compressibility in Avicel PH 301 with the “half-pressure” value of 151.449 MPa.

The study was financially supported by research project MSM 0021620822.

Address for correspondence:

Assoc. Prof. RNDr. Milan Řehula, CSc.

Univerzita Karlova v Praze, Farmaceutická fakulta v Hradci Králové, Katedra farmaceutické technologie

Heyrovského 1203, 500 05 Hradec Králové

e-mail: rehula@faf.cuni.cz