The optical method of measurement of sizes and the refractive index of nanoparticles Ag@Fe₃O₄

Overview

The spectrophotometric method and the electron microscope have been used to determine the distribution function of Ag@Fe₃O₄ nanoparticles by sizes and to measure their complex refractive index. These particles have been synthesized as acomponent of magnetically controlled drugs with antibacterial properties. The algorithm of processing the results of the experiment has been designed to measure the sizes of nanoparticles (1–100 nm).

Key words:
nanoparticle • sizes • measuring • spectrum • attenuation

Introduction

When solving various problems in physics, chemistry, biology, ecology and engineering, the size and optical properties of solid or liquid particles should be determined. Aerosols and water droplets in the atmosphere, emulsions and suspensions in liquids, bacteria, biological cells, etc., can be such particles.

To date the methods of microscopy^{1, 2)}, sedimentation³⁾ and others are used for solving these problems.

The optical methods are the most common ones^4–8). The optical beam is directed to the test volume containing particles. A photodetector records the parameters of the passed or diffused light such as its intensity, phase, and polarization. The mathematical processing of the signal from the photodetector allows determining parameters of particles^9–11).

The optical methods for determination of particle parameters can be divided into two large groups. In one group the indicatrix shape and the polarization parameters of the scattered particle radiation are measured, in another group the spectral dependence of the intensity of radiation transmitted through a medium is studied¹²⁾. To measure the parameters of nanoparticles, the latter methods find greater application. Their advantages are less sensitivity to the shape of particles than in the methods associated with the measurement of scattering indicatrix and the ability to measure dimensions of very small particles (with the radius of several nanometers). The mathematical basis of the methods is the Bouguer‘s law:

I = I₀e^-αl                                                                        [1]

where I – is the intensity of the light transmitted through a medium containing absorbing and scattering particles, I₀ – is the intensity of the incident light, l – is the thickness of the layer containing particles, α – is the attenuation coefficient.

If the environment contains N identical particles per unit volume, then neglecting the effect of multiple scattering of radiation (αl < 15) the attenuation coefficient is determined as follows:        

α (r, m, N, λ) = NQ(r, m, λ)πr²                                   [2]

where Q – is the efficiency factor of attenuation. The attenuation coefficient depends on the particle size r, their complex refractive index m = n – iκ, and the radiation wavelength λ.

If distribution of particles in size is described by the function f(r), then:

By measuring the dependence of the attenuation coefficient on the wavelength and knowing the form of the function Q(κ, r,m,λ) the average particle size can be determined using formula [2], whereas when using formula [3] the function of their distribution by size can be found.

Experimental Part

Materials and methods

The object of the measurements was the suspension of Ag@Fe₃O₄ nanoparticles obtained by the original single-phase method of chemical co-precipitation in water^{13, 14)}.

UV-transmission spectra were recorded with a SPEKOL-11 spectrophotometer (Carl Zeiss Jena, Germany) in cells with the layer thickness of 10 mm. The material of cells was colorless optical glass K-8; the wall thickness, 3 mm.

Experiment

Using a spectrophotometer, the transmission spectrum of the cell with a 1 mL suspension (obtained as the result of synthesis^{13, 14)}) was measured in the wavelength range of λ₀ = 0.400–0.840 µm (in the air). According to the formula

                     

which follows from the ratio (l), the attenuation coefficient was calculated. Here T = l/l₀ – is the luminous transmittance by the cell.

Figure 1 presents the graph of one of the dependences α(λ) where the abscissa axis shows the wavelengths of radiation in water.

Results and discussion

Data processing was carried out in three stages.

1. Measurement of the average particle size by the method of the power function

Based on the theory of light scattering by small particles¹⁵⁾, the dependence α(λ) is known to be described by the power function:

where А – is the coefficient depending on the particle concentration, size and the refraction index. The index of power q depends on the ratio between the particle radius r and the wavelength of light λ. If r<<λ and the particles are nonabsorbing, then q = 4 (Rayleigh law). Increasing the particle size, the value q decreases. For very small absorbing particles q = 1 and when increasing the particle size, at first the value q increases, and then it decreases.

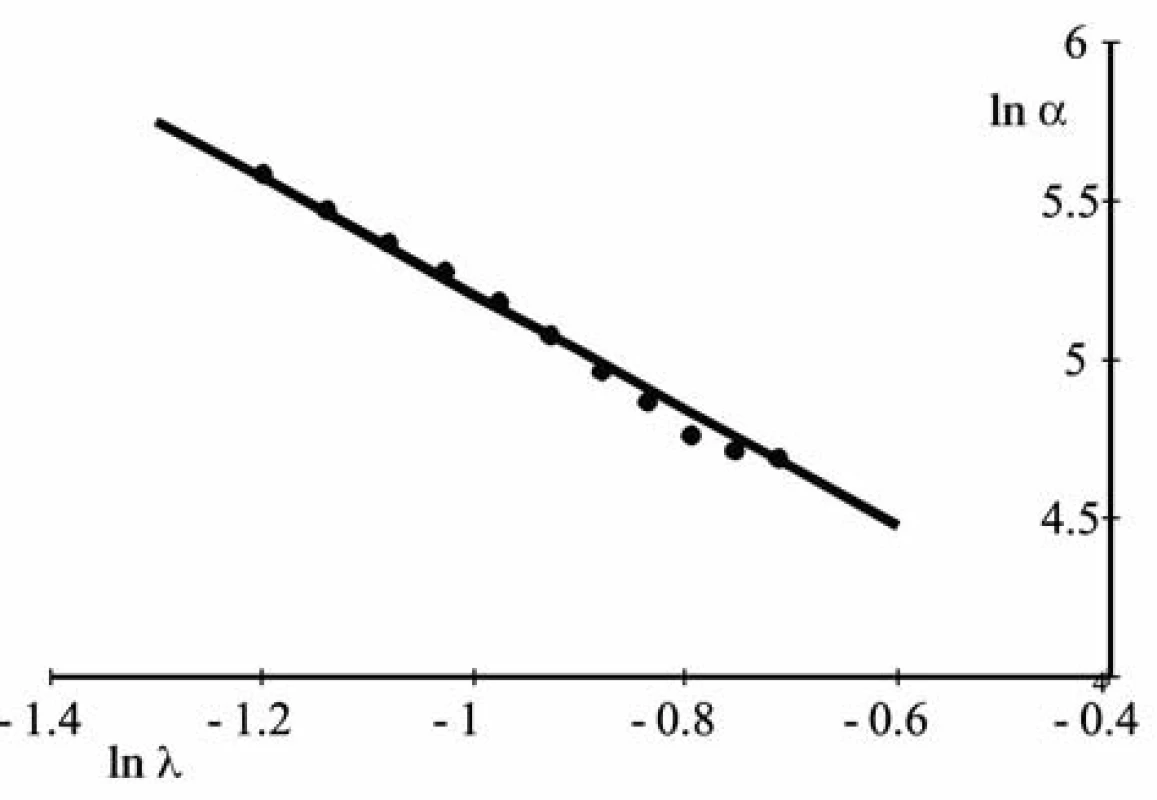
The index of power q can be determined from experimental data as follows. After taking the logarithm of formula (5), one can get a linear dependence (Fig. 2).

lnα = lnA – qlnλ.                                                       [6]

The value q here is the angular coefficient. It can be found using the least square method.

Combining formulas [2] and [5] we find that:

where ρ = πd/λ.

This equation relates the values q and ρ. Having determined the index of power q, it is possible to find the value ρ and particle sizes. When calculating the function Q(m,ρ), one can use formulas for the sphere^16–18), which give good results in the case of light scattering on the arrays of randomly distributed particles of the irregular shape:

where

ψ₁(z) and ζ₁(z) – are Bessel- Riccati functions, the sign′ means derivative of the function throughout the argument.

For calculations one should know the complex refractive index of the particle substance. The nanoparticles of magnetite and silver are present in the suspension. According to the literature data^19–20), their refractive indices in the air at the wavelength of 0.5 μm are as follows:

magnetite – m₀ = 2 – 0.04i                                        [11]

silver – m₀ = 0.05 – 3.09i                                            [12]

The calculations by formulas [8–10] show that efficiency of the attenuation coefficient for silver nanoparticles is much greater than in magnetite nanoparticles; therefore, mainly these particles affect scattering and absorption of light in the cell. Hence, the value of the refractive index [12] should be used in calculations by formula [6].

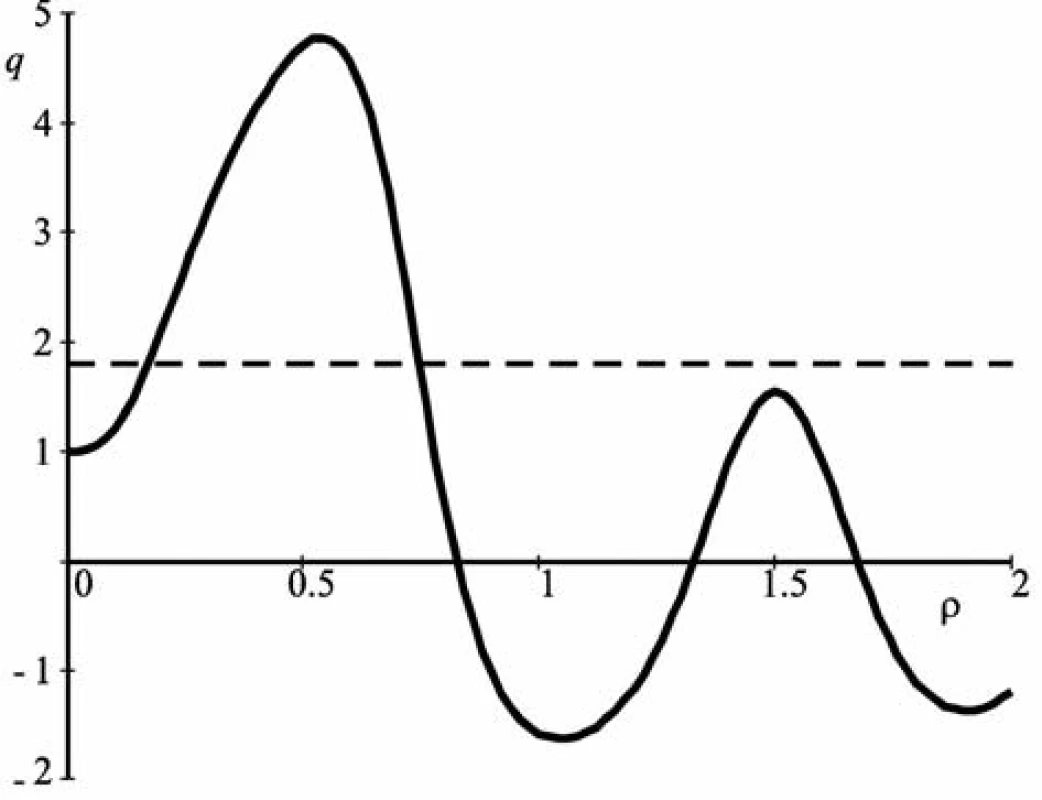
The graph of q(ρ) function calculated according to these data is shown in Figure 3.

The horizontal dashed line is drawn at the level of the value q = 1.825. Two values of the parameter ρ correspond to it: ρ₁ = 0.18 and and ρ₂ = 0.74.

The value ρ₂ should be omitted since the value of the particle size corresponding to it is not found in the nanometer range since it is in the micrometer range.

The particle diameter is determined by the ratio: d = ρλ_av/π = 27 nm.

The value of the average wavelength in the working range λ_av = 470 nm was used.

2. Measurement of parameters of particles by the method of spectral transparency

The idea of the method consists in selecting such values of the parameters N, r, n, κ in equation [2] when the theoretical dependence α(λ) coincides well with the experimental one. The function type Q (r, n, κ, λ) must be known. For this purpose formulas [6–8] are used.

The number of unknowns in formula [2] is four. Their number can be reduced by using the attenuation values αα_i and αα_j at wavelengths λ_i and λ_j, as well as dividing equation [2] one upon the other:

To find the unknowns r, n, κ, it is sufficient to take three equations [13]. The following values of dependence α(λ) obtained in the experiment were used (see Fig. 1):

  To solve the resulting system of equations, the MATHCAD program was used. As the initial approximations, the values obtained above were taken: r = 13.5 nm, n = 0.05, κ = 3.09.

The results of the solution are as follows: r = 17.5 nm, n = 1.65, κ = 5.11.

Such values of the parameters obtained indicate that the value of the particle radius by the previous method has been found to be close to the true value. The differences may be due to the fact that when calculating the particle size the average value of the wavelength λ_av = 470 nm was used. From the graph in Figure 2 it is seen that when using another spectral range (e.g., from 500 to 700 nm) there would be other values λ_av, and the particle size would be different. This is a drawback of the previous method. But it is suitable to determine the initial value of the particle size when solving the system of equations [13].

The values of the optical constants obtained (n = 1.65, κ = 5.11) show that the refractive index n is determined by magnetite particles, and the absorption index, by silver particles. For air, these values are as follows: n₀ = 2.19, κ₀ = 6.80

Using formula [2], the concentration of particles in the medium is determined:

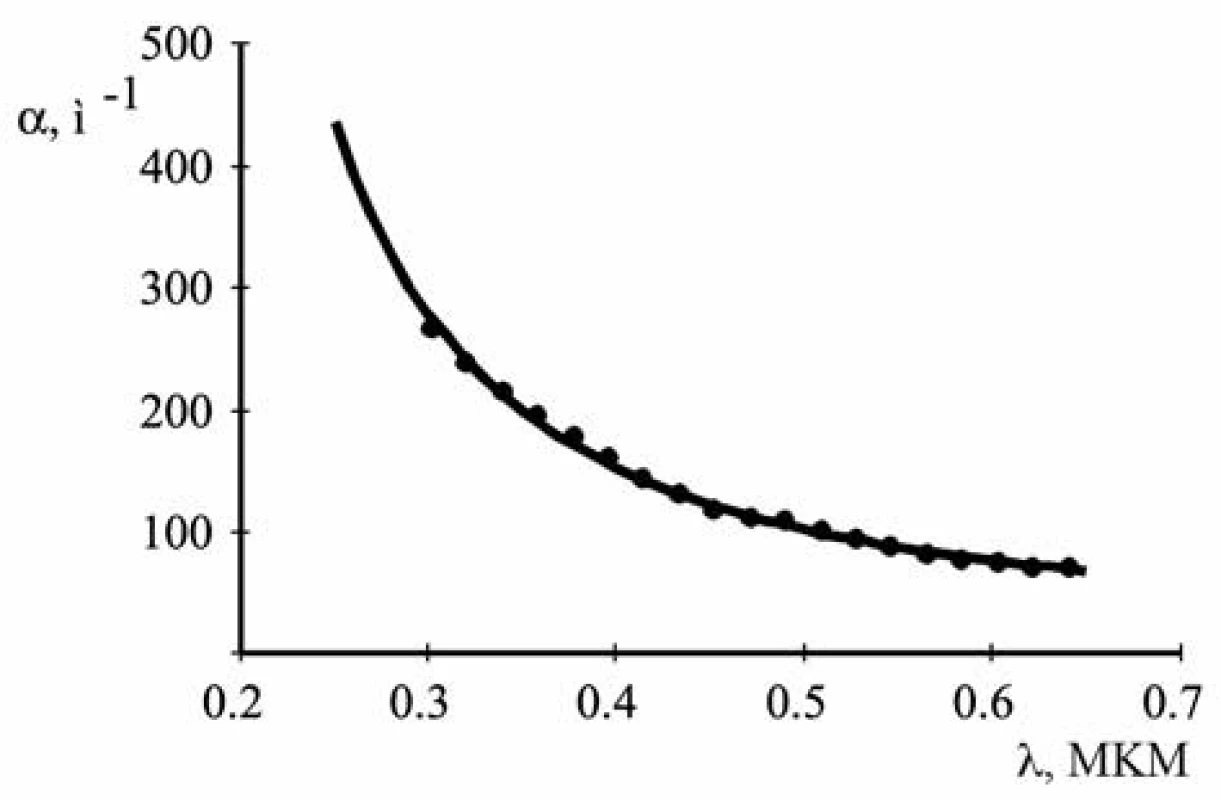
Figure 4 shows the curve calculated by formula [2] using the values r, n, κ, N obtained. It is apparent that it passes through the array of experimental points. This suggests that these values are close to true values.

3. Measurement of the function of particle size distribution

In order to determine the function of particle size distribution f(r) the integral equation [3] should be solved. This is Fredholm equation of the first kind. It is very sensitive to errors in the initial data – the function α(λ)²¹⁾. Therefore, when solving it the method of parameterization is often used – the formula for the function f(r) is given, and the values of its parameters, which the calculated function α(λ) most closely corresponds to the experimental results, are found.

The disadvantage of the method of parameterization is the need for a priori assignment of the distribution function f(r). However, often the error in the solution of the integral equation [3] in general is so great that it makes equal the validity of the results obtained by those methods and the method of parameterization.

The function of particle size distribution was given in the form of the Gaussian function:

Here r_m is the most probable the value of the particle radius. The standard deviation Δr characterizes the value of particle size distribution.

Such function according to the literature data⁹⁾ describes well the distribution of particles with sizes in the range from 0.01 to 100 μμm and a small variation in size.

The attenuation coefficient α was calculated as the integrated sum by the method of trapezoids with a fixed number of intervals – 40.

Parameters r_m, Δr and N were determined by the least square method. For this purpose the minimum of the function was found:

where α_i – are the experimental values of the attenuation coefficient at wavelengths λ_i.

The initial values of parameters r_m, Δr, N were set as those obtained at the second stage of calculations.

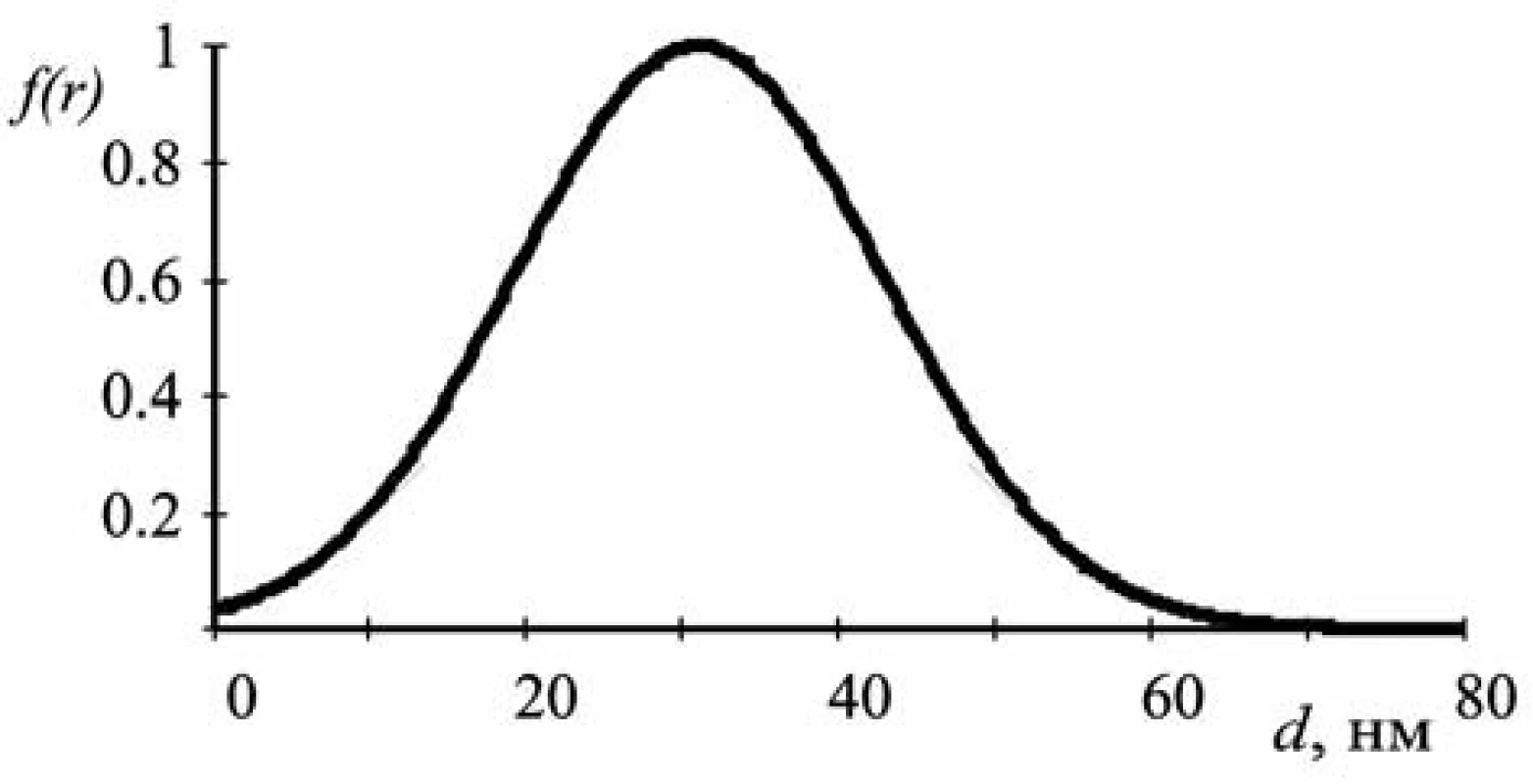
The following parameters of the function f(r) were obtained: r = 17 nm, Δr = 8 nm.

The graph of the function of particle size distribution is given in Figure 5. The abscissa axis shows the values of the particle diameter in nanometers, the ordinate axis demonstrates the values of the function f(r) normalized to the maximum value.

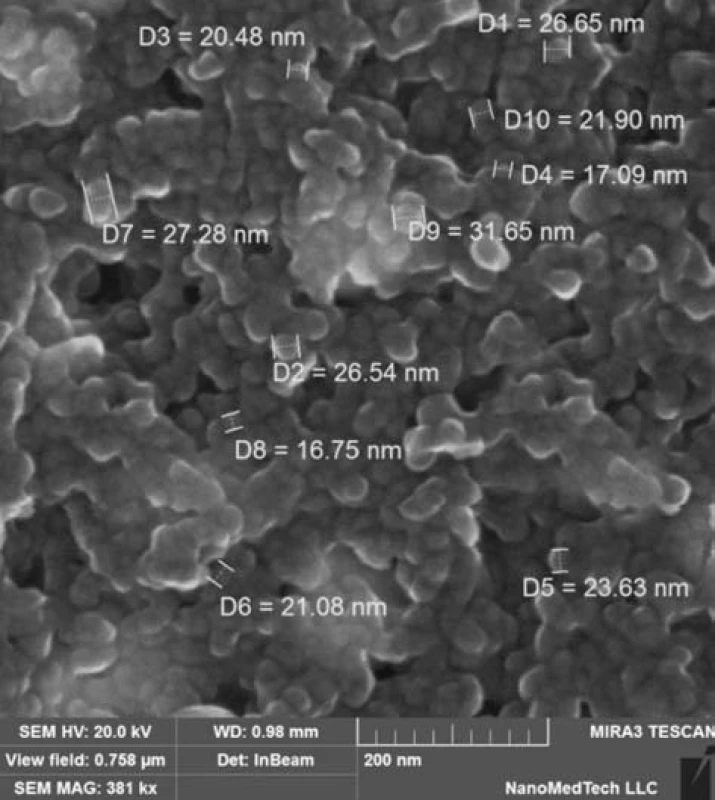
The data obtained by the computational methods proposed are in good agreement with the results of similar studies by electron microscopy (Fig. 6)^13–15).

Conclusions

Methods of measurement of size and optical properties of Ag@Fe₃O₄ nanoparticles based on the treatment of the light attenuation spectrum (such as determining the average particle size by the method of the power function, measuring the parameters of particles by the method of spectral transparency and the function of particle size distribution) give results consistent with the observations of particles using an electron microscope.

Conflicts of interest: The authors have declared that there are no financial relationships with any organizations that might have an interest in the submitted work, nor any other relationships or activities that could appear to have an influence on the submitted work.
Conflict of interest: none.

Received April 7, 2016 / Accepted May 31, 2016

Mykola G. Kokodiy

National University of Pharmacy

53, Pushkinska st., 61002 Kharkiv, Ukraine

V. N. Karazin Kharkiv National University

4, Svobody Sq., 61022 Kharkiv, Ukraine

e-mail: Kokodiy.N.G@gmail.com